Thoughts on Conceptual Understanding

In a previous post, I talked about different beliefs held by math teachers and others regarding when the standard algorithm for multi-digit addition and subtraction should be taught. Some believe that it should be taught only after students have had experience and practice with alternative place-value strategies. The reason is that such strategies provide the conceptual understanding that underpins the standard algorithm, which it is further believed eclipses these concepts. Others believe that it should be taught first, concurrent with the concepts that inform the procedure.

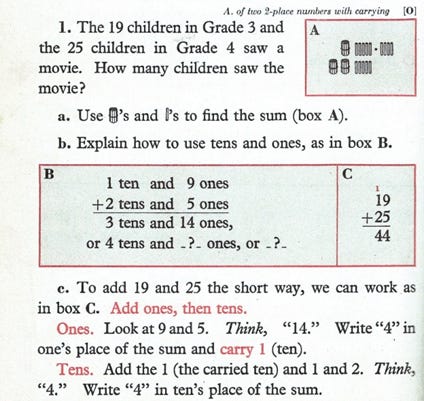
Teaching the standard algorithm first does not mean that it is taught without connection to the underlying principles that make it work. In fact, the connection between place value and the algorithm itself can be taught concurrently as it has been done in traditional math teaching. Older textbooks did in fact show what is being done mathematically when using the standard algorithm as seen in the two figures below, taken from the textbook “Arithmetic We Need” by Buswell, Brownell and Sauble (1955). Figure 1 shows the method for adding two two-digit numbers without carrying (or “regrouping” as it is currently called).

Figure 1 (Brownell, et al; 1955)

Students are first shown sticks in bundles of tens, and single ones, to represent the numbers 32 and 13 — and they are asked to complete the addition. (The teacher may in fact present what is in the figure independently, asking students the same questions as are in the text.) The next picture has the numbers written out in terms of “tens” and “ones”, illustrating place value; again. As before, students are asked to finish the calculation. Finally, Box C connects the previous two pictures to the procedure for the standard algorithm which is characterized as a “short way” of doing what was shown in the previous pictures. Prior to this lesson, students have worked with stick bundles before, and they have also been introduced to the concept of place value.

Figure 2 below shows similar diagrams, this time illustrating how numbers in the ones column are “carried” over to the tens column when the sum is greater than or equal to ten.  As in Figure 1, the standard algorithm is characterized as the “short way” of doing what the place value strategies represented in Boxes A and B.

Figure 2 (Brownell, et al; 1955)

I can attest that when I first learned this (in second grade) I wasn’t that interested in the explanation, other than the fact that it made a “kind of sense” and was a lot easier than drawing sticks to count up by the number being added. I was happy to be able to do the procedure. When we learned about carrying I was then able to make the connection with the concept of place value.

Procedural fluency is based upon foundational contexts within which conceptual understanding is developed and explained. As one goes on in math, the contexts for various procedures become more abstract, and the concepts have more procedural connections associated with them. A good example of this is the topic of division, which starts with whole number division, and then moves on to fractional division. Students in high school will generally have the tools and connections to procedures to understand the underlying concept for fractional division. Thus a ÷ b is defined as a/b = a · 1/b where b does not equal zero. This is the general concept of division that works for integers as well as for fractions. Reaching this level of understanding depends on a student knowing and mastering various procedures and concepts such as: a) 24 ÷ 3 is the same as 1/3 of 24 or 1/3 · 24, b) that a/b · c/d = ac/bd, c) that a/b · b/a = 1, and therefore 1÷(a/b) = 1/(a/b) = b/a, and other foundational procedures that are built upon over time.

It is important to note, however, that for some students there may be certain procedures for which the concept will come later (as it did for me). Also there will be concepts that some students will never fully understand. The idea that one hundred percent of all students must understand one hundred percent of all the concepts behind one hundred percent of all procedures is one hundred percent unrealistic, misguided and damaging. What is key is connecting the procedure to the context in which it is used, and the math it represents. Also key is that a student recognize what is needed to solve a problem, and execute the procedures necessary to solve it.

Reference:

Brownell, William A., Buswell, Guy T., Sauble, Irene; 1955; “Arithmetic We Need, Grade 3”; Ginn and Company, New York.